
It is a beautiful Spring morning. You are waiting in line to get your picture taken with a man in an Easter Bunny costume, as an amusing gift for your friends. When you get to the head of the line, the bunny says, “My! You are a very big child.”
“Oh!” you laugh, “I am not a child. I am just doing this as an amusing gift for my friends. I am actually a calculus student.”
“What a sweet and precocious word to use, ‘calculus’, did you learn that word from your older sister or brother?”
“No,” you protest. “I really AM a calculus student. Why, just yesterday I learned all about Taylor series.”
“Taylor series? How cute! That is… if you think it is cute to learn about LIES!” The person in the Easter Bunny costume is none other than your wild-eyed hungry looking tormentor!
While you are frozen on his lap in horror, he asks you, “Please tell me, if you will, what is the solution to this integral?
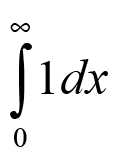
Out of sheer reflex you answer his simple question. “The integral diverges. It doesn’t go to any finite number.”
“Bad person being mean to Easter Bunny!” says the little girl in line behind you.
The stranger ignores her. “How about these two?”
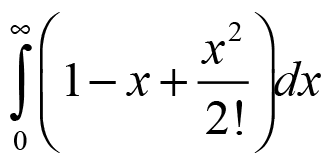
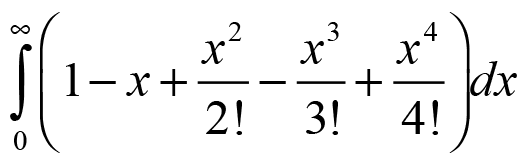
Leaving aside the question of how he can talk in math notation, you think for a moment, and then say “They both diverge. In fact, any polynomial like that is going to diverge, going off to infinity. It doesn’t matter what the denominators of the coefficients are.”
“So you are saying that; no matter how large k gets, this is true?”

“Well… yes.”
Several angry children are throwing chocolate eggs at you. He continues to talk in math notation: “But

and that would make
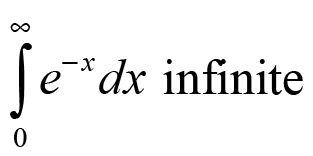
But

So infinity is equal to one! Now get off my lap!” As hundreds of children “Boo” you, you walk away, thinking about that integral. The faux Easter Bunny has just proved that one is equal to infinity! Can this be true? Does it no longer “take one to know one”? Does this mean that all monotheists have suddenly become pantheistic? Or is there a chance, however small, that our hare-y friend has made a mistake? Find the error.
Find the error.
- Find the error: Differentiation
- Find the error: L’Hopital’s Rule
- Find the error: Related Rates
- Find the error: Fundamental Theorem of Calculus
- Find the error: U-substitution
- Find the error: Integration by Parts (part 1)
- Find the error: Integration by Parts (part 2)
- Find the error: Trigonometric Integration
- Find the error: Taylor Series
- Find the error: Improper Integrals and Taylor Series
- Find the error: Separation of Variables
- Find the error: Separation of Variables – Exponential Growth
- Find the error: Solutions
- Find the error: Credits